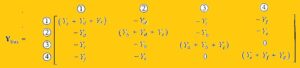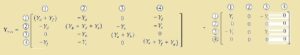Hello, readers welcome to new post. In this post, we will learn how to solve node equations. Node equation re technique that used to solve different circuits in the electrical power system in a simple way. It makes our work easy and simple related to find the parameters used in the system like volts current and power.
So let get started
How to Solve Node Equation
- The common point in the circuitry where two elements of circuitry are linked is called a node.
- Normally KCL and KVL are used to solve the different equations existing in the circuitry.
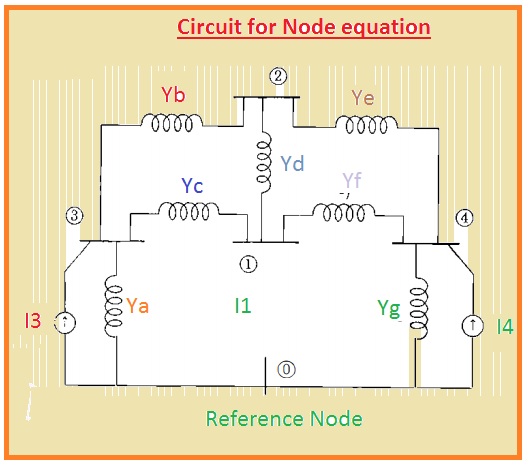
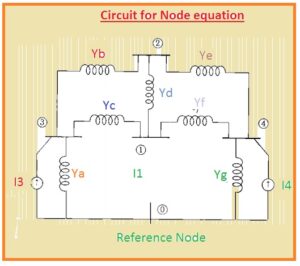
- To the understanding of node, equation let’s take a circuitry and solve it.
- In this circuitry, nodes are denoted with a number in the circle.
- Current sources are linked at nodes number three and four and other elements are taken as admittance.
- This is the usage of single subscript notation to make the voltage values of every node according to the reference node
- If we apply KCL at node number one current is moving away from the node so the equation will be
(V1-V3)Yc+(V1-V2)Yd+(V1-V4) Vf=0
- At not number three we have
V3Ya-(V3-V2)Yb+(V3-V1)Yc=I3
- After simplifying this expression we have
- For node number one
V1(Yc+Yd+Yf)-V2Yd-V3Yc-V4Yf=0
- For node three
-V1Yc-V2Yb+V3(Ya+Yb+YC)=I3
- Like this equation can be designed for nodes two and four and this equation can be resolved to find the values of volts
- By using the values of volts V1, V2 V3, and V4 current in all branches of circuitry can be determined
- Now we write the above four equations in matrix form and we have.
- this form of the equation helps us to easily solve them and the parameters of every node also remain in the mind.
- the matrix that we form is denoted as Ybus and known as bus admittance matrix.
- there are some rules you must follow to make Ybus admittance.
- All the diagonal components Yii is equal to the addition of admittance linked to the node.
- The components that are not in diagonal equal to the negative of total admittance linked among the nodes i and j
- the diagonal admittance is known as self admittance mutual admittance is known as mutual admittance.
- after the application of Ybus rules, we have a new matrix
- The number in the circle denotes the number of nodes.
- Let us take components for Yc admittance that we have
- this matrix can also be defined as
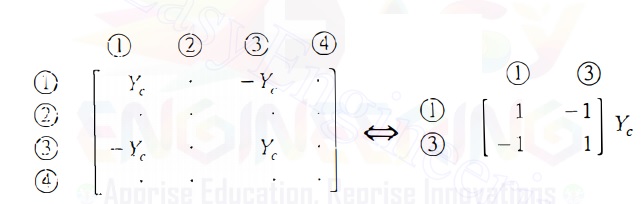
- now we transform this admittance matrix to an impudence matrix so we have
that is all bout the How to Solve Node Equations i have solved detailed circuitry for you to understand node equation if you have still any queries ask in the comments thanks for reading have a nice day.
